In our latest math project, we explored linear equations. More specifically though, we looked at rising and lowering water levels. We split up into partners and I worked with Morgan to research the impact of climate change on the Dead Sea. The Dead Sea, located between Israel and Jordan, has been drying up at a very rapid rate for hundreds of years.
Driving question:
How much area (of water) does the Dead Sea lose every year and how long would it take for it to lose all of its area?
Information We Started With
– The current area of the Dead Sea is 605km^2
-In 1930 it’s area was 1050km^2
-Meaning, 445km^2 of area was lost in 88 years
Our Equation
Using this information, we calculated the rate of change using a slope equation. In this equation,
Y = Area lost
X = Years
M = Rate of change (Area lost over years)
B = amount lost to start (In this case, 0)
Therefore, our equation is Y = 445/88 X + 0
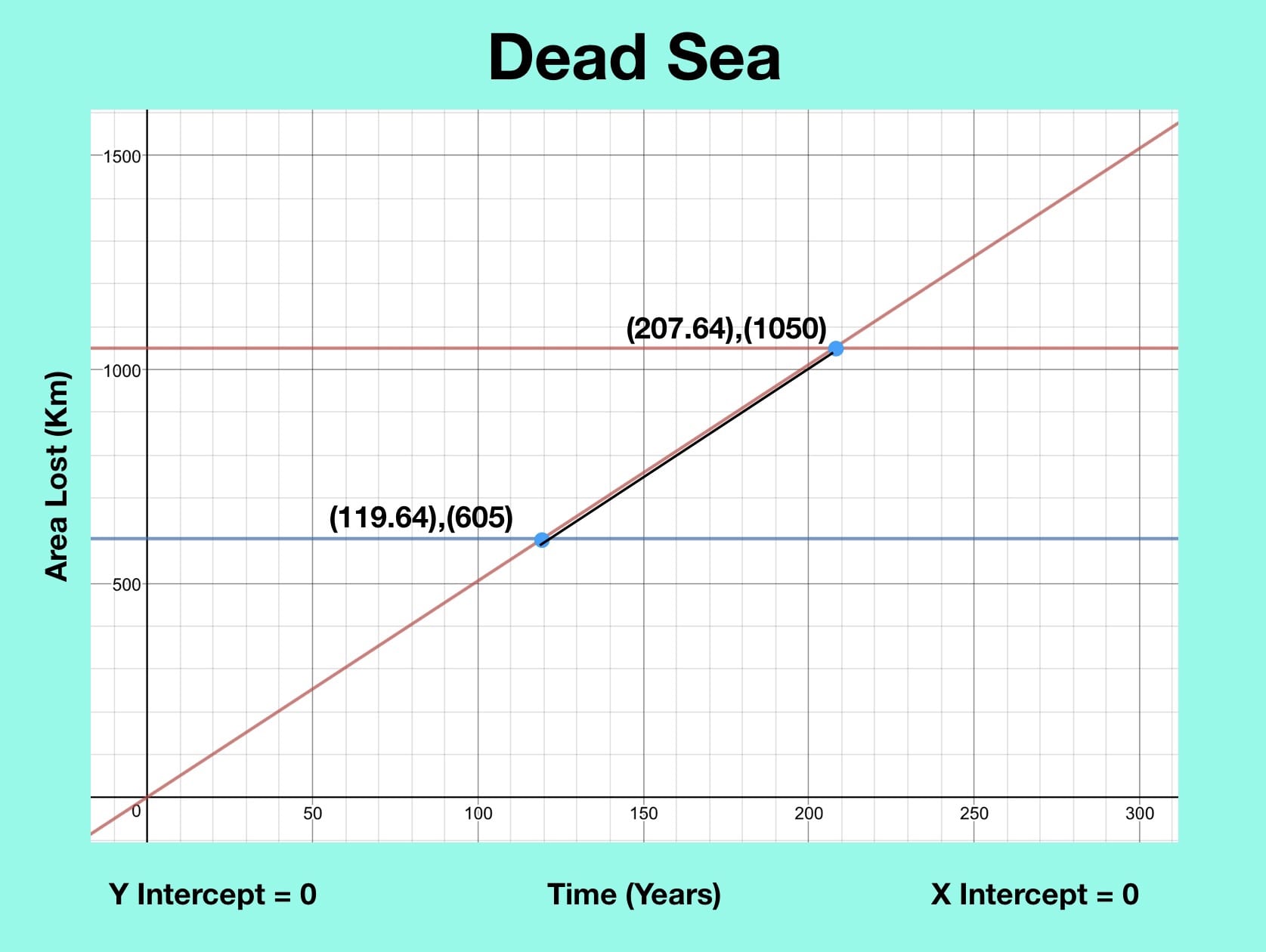
Our Graph
Why is This Happening?
This is happening for a couple of reasons. First of all, the Dead Sea has a very high salt content, which causes it to be dryer than normal seawater. Second, and more importantly, climate changed has caused more and more water to evaporate at a very fast rate.
In Conclusion
-To lose 1050 km^2 ( the original amount) it would take ~207.64 years
-To lose 605 km^2 (the current amount) it will take ~ 119 years
-This means that it loses around 5.057 km^2 per year
Competencies I used




Project End Mind Map